Aligned Resources

Outlines the approach to building fractals by cutting out portions of plane figures.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: area, distance, fractals, fractions, generator, geometric sequences, geometry, infinity, initiator, iteration, length, limit, lines, multiplication, pattern, percents, perimeter, recursion, scale, segment, self-similarity, sequences, sets

Explores lines, planes, angles, and polygons in tessellations.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: angles, area, flips, geometry, glides, hexagon, length, lines, pattern, perimeter, planes, polygon, rectangles, reflections, regular, rotation, slides, squares, symmetry, tessellations, triangle

Introduces students to the idea of finding number patterns in the generation of several different types of fractals.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: area, arithmetic, chaos, decimals, dimension, fractals, fractions, geometric sequences, geometry, graph, iteration, length, lines, pattern, pythagorean theorem, rectangles, recursion, segment, self-similarity, sequences, surface area, symmetry, triangle

A capstone lesson to allow students to build a working definition of fractal.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: area, chaos, dimension, distance, experimental probability, exponents, fractals, fractions, generator, geometric probability, geometric sequences, geometry, infinity, initiator, iteration, length, limit, lines, logarithm, multiplication, outcomes, pattern, percents, perimeter, probability, random number, recursion, scale, segment, self-similarity, sequences, sets, theoretical probability, triangle

Students learn how the Pythagorean Theorem works and how to apply it.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry, Trigonometry

Related Topics: algebra, angles, area, arithmetic, cartesian coordinate, coordinate plane, distance, exponents, geometry, hypotenuse, length, perimeter, pythagorean theorem, right angle, slides, solving equations, squares, triangles, trigonometry

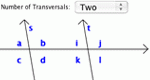
Practice your knowledge of acute, obtuse, and alternate angles. Also, practice relationships between angles - vertical, adjacent, alternate, same-side, and corresponding. Angles is one of the Interactivate assessment explorers.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: acute, angles, assessment, geometry, lines, obtuse, parallel

Students work step-by-step through the generation of a different Hilbert-like Curve (a fractal made from deforming a line by bending it), allowing them to explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: decimals, fractals, fractions, geometric sequences, geometry, iteration, length, pattern, self-similarity, sequences, surface area, symmetry

Learn about fractions between 0 and 1 by repeatedly deleting portions of a line segment, and also learn about properties of fractal objects. Parameter: fraction of the segment to be deleted each time.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: fractals, fractions, geometric sequences, pattern, pre-calculus, recursion, sequences, sets

Create your own fractals by drawing a "line deformation rule" and stepping through the generation of a geometric fractal. Parameters: Grid type, number of bending points on the line.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Geometry, Number and Operations

Related Topics: chaos, fractals, geometric sequences, iteration, pattern, pre-calculus, recursion, scale, self-similarity, sequences, symmetry, transformation

Step through the generation of a Hilbert Curve -- a fractal made from deforming a line by bending it, and explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: chaos, fractals, geometric sequences, geometry, iteration, length, lines, pattern, recursion, self-similarity, sequences

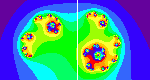
Enter a complex value for "c" in the form of an ordered pair of real numbers. The applet draws the fractal Julia set for that seed value.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: complex number, coordinate plane, coordinate system, fractals, iteration, julia set, mandelbrot set, recursion, recursive functions, sets

Step through the generation of the Koch Snowflake -- a fractal made from deforming the sides of a triangle, and explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: decimals, fractals, geometric sequences, infinity, iteration, lines, pre-calculus, recursion, self-similarity, sequences

Calculate the length of one side of an automatically generated right triangle by using the Pythagorean Theorem, and then check your answers. Pythagorean Explorer is one of the Interactivate assessment explorers.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry, Trigonometry

Related Topics: algebra, angles, assessment, distance, exponents, geometry, length, pythagorean theorem, solving equations, squares, triangle, trigonometry

Step through the generation of Sierpinski's Carpet -- a fractal made from subdividing a square into nine smaller squares and cutting the middle one out. Explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: algebra, area, fractals, fractions, geometric sequences, iteration, pattern, pre-calculus, recursion, recursive functions, self-similarity, sequences

Step through the generation of Sierpinski's Triangle -- a fractal made from subdividing a triangle into four smaller triangles and cutting the middle one out. Explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: dimension, fractals, fractions, geometric sequences, geometry, iteration, length, pattern, pre-calculus, recursion, recursive functions, self-similarity, sequences, symmetry, triangle

Learn about how the Pythagorean Theorem works through investigating the standard geometric proof. Parameters: Sizes of the legs of the triangle.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry, Trigonometry

Related Topics: algebra, angles, area, coordinate plane, distance, geometry, length, pythagorean theorem, slides, solving equations, squares, triangle, triangles, trigonometry

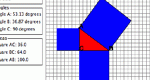
Calculate the area of a triangle drawn on a grid. Learn about areas of triangles and about the Cartesian coordinate system. Triangle Explorer is one of the Interactivate assessment explorers.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: acute, area, assessment, cartesian coordinate, coordinate plane, distance, geometry, length, pythagorean theorem, triangle, triangles