Aligned Resources

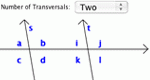
Introduces students to acute, obtuse, and right angles as well as relationships between angles formed by parallel lines crossed by a transversal.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: acute, adjacent, alternate exterior, alternate interior, angles, corresponding, geometry, intersection, lines, obtuse, rays, right angle, transversal, vertical

This lesson utilizes the concepts of cross-sections of three-dimensional figures to demonstrate the derivation of two-dimensional shapes.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: conic section, geometry, hyperbola, parabola, polygon, pre-calculus, pyramid

Outlines the approach to playing the chaos game and how it relates to geometric fractals.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Number and Operations, Probability

Related Topics: chaos, experimental probability, fractals, fractions, geometric probability, infinity, iteration, outcomes, pattern, percents, probability, probability simulation, random number, recursion, self-similarity, theoretical probability, triangle

Outlines the approach to building fractals by cutting out portions of plane figures.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: area, distance, fractals, fractions, generator, geometric sequences, geometry, infinity, initiator, iteration, length, limit, lines, multiplication, pattern, percents, perimeter, recursion, scale, segment, self-similarity, sequences, sets

Explores lines, planes, angles, and polygons in tessellations.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: angles, area, flips, geometry, glides, hexagon, length, lines, pattern, perimeter, planes, polygon, rectangles, reflections, regular, rotation, slides, squares, symmetry, tessellations, triangle

Introduces students to the ideas involved in understanding fractals.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: area, distance, fractals, fractions, generator, geometric sequences, geometry, infinity, initiator, iteration, length, limit, lines, multiplication, pattern, percents, perimeter, recursion, scale, segment, self-similarity, sequences, sets

Looks at how irregular fractals can be generated and how they fit into computer graphics.

Audiences: Grades 9-12, Undergraduate

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: chaos, dimension, fractals, geometric sequences, geometry, iteration, logarithm, pattern, planes, polygon, recursion, scale, self-similarity, symmetry

Introduces students to lines, rays, line segments, and planes.

Audiences: Grades 3-5, Grades 6-8

Primary Subjects: Geometry

Related Topics: algebra, axis, coordinate plane, coordinate system, functions, geometry, graph, infinity, lines, number line, planes, rays, segment

Introduces students to the idea of finding number patterns in the generation of several different types of fractals.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: area, arithmetic, chaos, decimals, dimension, fractals, fractions, geometric sequences, geometry, graph, iteration, length, lines, pattern, pythagorean theorem, rectangles, recursion, segment, self-similarity, sequences, surface area, symmetry, triangle

Students learn about how probability can be represented using geometry.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Probability

Related Topics: angles, area, circle graph, circles, counting, decimals, degrees, estimation, events, experimental probability, fair, geometric probability, geometry, length, outcomes, percents, polygon, polyhedra, probability, probability simulation, random number, right angle, spinner, statistics, theoretical probability, volume

A capstone lesson to allow students to build a working definition of fractal.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: area, chaos, dimension, distance, experimental probability, exponents, fractals, fractions, generator, geometric probability, geometric sequences, geometry, infinity, initiator, iteration, length, limit, lines, logarithm, multiplication, outcomes, pattern, percents, perimeter, probability, random number, recursion, scale, segment, self-similarity, sequences, sets, theoretical probability, triangle

Introduces students to the concepts of surface area and volume.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: area, arithmetic, dimension, geometry, integers, length, polyhedra, prisms, surface area, volume

This lesson teaches students how to find the surface area of non-rectangular prisms.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: area, dimension, geometry, length, polyhedra, prisms, rectangles, slant height, surface area, triangle, volume

This lesson teaches students how to find the surface area of rectangular prisms.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: area, dimension, geometry, length, polyhedra, prisms, rectangles, surface area

Examines plane symmetry.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: angles, area, geometry, glides, hexagon, illusion, length, pattern, planes, polygon, rectangles, reflections, regular, rotation, segment, squares, symmetry, tessellations, transformation, translation, triangle

Introduces all of the 2 variable function and prisoner/escapee notions necessary to understand the Mandelbrot set.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: chaos, complex number, coordinate, coordinate plane, coordinate system, division, escape, exponents, fractals, functions, geometric sequences, geometry, infinity, iteration, julia set, mandelbrot set, multiplication, pattern, planes, prisoner, radius, recursion, recursive functions, self-similarity, sets, symmetry

This lesson teaches students how to find the volume of non-rectangular prisms.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: area, depth, dimension, geometry, height, length, multiplication, polyhedra, prisms, rectangles, slant height, squares, surface area, triangle, volume, width

This lesson teaches students how to find the volume of rectangular prisms.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: area, depth, dimension, geometry, height, length, multiplication, polyhedra, prisms, rectangles, squares, triangle, volume, width

Practice your knowledge of acute, obtuse, and alternate angles. Also, practice relationships between angles - vertical, adjacent, alternate, same-side, and corresponding. Angles is one of the Interactivate assessment explorers.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: acute, angles, assessment, geometry, lines, obtuse, parallel

Students work step-by-step through the generation of a different Hilbert-like Curve (a fractal made from deforming a line by bending it), allowing them to explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: decimals, fractals, fractions, geometric sequences, geometry, iteration, length, pattern, self-similarity, sequences, surface area, symmetry

Learn the relationship between perimeter and area. A shape will be automatically generated with the perimeter that you choose. Calculate the area of this shape. Area Explorer is one of the Interactivate assessment explorers.

Audiences: Grades 3-5, Grades 6-8

Primary Subjects: Geometry

Related Topics: addition, area, arithmetic, assessment, comparing, coordinate plane, geometry, length, perimeter, polygon, rectangles, squares

Explore cross sections of different geometric solids: cone, double cone, cylinder, pyramid, and prism. Manipulate the cross section with slider bars, and see how the graphical representation changes.

Audiences: Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus, Geometry, Graphs

Related Topics: algebra, calculus, cartesian coordinate, circles, conic section, coordinate system, ellipse, flyer, graph, hyperbola, parabola, polygon, polyhedra, pre-calculus, prisms, pyramid, radius, rotation, transformation

Build a "floor tile" by dragging the corners of a quadrilateral. Learn about tessellation of quadrilateral figures when the shape you built is tiled over an area.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: angles, area, coordinate plane, coordinate system, length, pattern, planes, quadrilaterals, rectangles, reflections, rhombus, rotation, slides, symmetry, tessellations, transformation, translation

Generate complicated geometric fractals by specifying starting polygon and scale factor.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Geometry

Related Topics: fractals, geometry, logarithm, polygon, recursion, scale, self-similarity

Step through the generation of a Hilbert Curve -- a fractal made from deforming a line by bending it, and explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: chaos, fractals, geometric sequences, geometry, iteration, length, lines, pattern, recursion, self-similarity, sequences

Enter a complex value for "c" in the form of an ordered pair of real numbers. The applet draws the fractal Julia set for that seed value.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: complex number, coordinate plane, coordinate system, fractals, iteration, julia set, mandelbrot set, recursion, recursive functions, sets

Step through the generation of the Koch Snowflake -- a fractal made from deforming the sides of a triangle, and explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: decimals, fractals, geometric sequences, infinity, iteration, lines, pre-calculus, recursion, self-similarity, sequences

Learn the relationship between perimeter and area. A shape will be automatically generated with the area that you choose. Calculate the perimeter of this shape. Perimeter Explorer is one of the Interactivate assessment explorers.

Audiences: Grades 3-5, Grades 6-8

Primary Subjects: Geometry

Related Topics: area, arithmetic, assessment, comparing, coordinate plane, coordinate system, geometry, length, perimeter, polygon, rectangles, squares

This activity operates in one of two modes: auto draw and create shape mode, allowing you to explore relationships between area and perimeter. Shape Builder is one of the Interactivate assessment explorers.

Audiences: Grades 3-5, Grades 6-8

Primary Subjects: Geometry

Related Topics: area, assessment, comparing, geometry, perimeter

Learn the relationship between perimeter and area. A random shape will be automatically generated. Calculate the area and perimeter of this shape. Shape Explorer is one of the Interactivate assessment explorers.

Audiences: Grades 3-5, Grades 6-8

Primary Subjects: Geometry

Related Topics: area, arithmetic, assessment, comparing, geometry, length, perimeter, polygon, rectangles, squares

Step through the generation of Sierpinski's Carpet -- a fractal made from subdividing a square into nine smaller squares and cutting the middle one out. Explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: algebra, area, fractals, fractions, geometric sequences, iteration, pattern, pre-calculus, recursion, recursive functions, self-similarity, sequences

Step through the generation of Sierpinski's Triangle -- a fractal made from subdividing a triangle into four smaller triangles and cutting the middle one out. Explore number patterns in sequences and geometric properties of fractals.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: dimension, fractals, fractions, geometric sequences, geometry, iteration, length, pattern, pre-calculus, recursion, recursive functions, self-similarity, sequences, symmetry, triangle

Learn about how the Pythagorean Theorem works through investigating the standard geometric proof. Parameters: Sizes of the legs of the triangle.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry, Trigonometry

Related Topics: algebra, angles, area, coordinate plane, distance, geometry, length, pythagorean theorem, slides, solving equations, squares, triangle, triangles, trigonometry

Manipulate dimensions of polyhedra, and watch how the surface area and volume change. Parameters: Type of polyhedron, length, width and height. Surface Area and Volume one of the Interactivate assessment explorers.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: assessment, dimension, geometry, length, polyhedra, prisms, surface area, volume

Create a tessellation by deforming a triangle, rectangle or hexagon to form a polygon that tiles the plane. Corners of the polygons may be dragged, and corresponding edges of the polygons may be dragged. Parameters: Colors, starting polygon.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: angles, area, flips, geometry, glides, hexagon, length, pattern, planes, polygon, reflections, rotation, slides, squares, symmetry, tessellations

Explore fractals by investigating the relationships between the Mandelbrot set and Julia sets.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Discrete, Fractions, Geometry, Number and Operations

Related Topics: chaos, complex number, coordinate, coordinate plane, exponents, fractals, functions, geometric sequences, geometry, iteration, julia set, mandelbrot set, pattern, recursion, recursive functions, self-similarity, sets

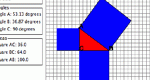
Calculate the area of a triangle drawn on a grid. Learn about areas of triangles and about the Cartesian coordinate system. Triangle Explorer is one of the Interactivate assessment explorers.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Geometry

Related Topics: acute, area, assessment, cartesian coordinate, coordinate plane, distance, geometry, length, pythagorean theorem, triangle, triangles

Enter two complex numbers (z and c) as ordered pairs of real numbers, then click a button to iterate step by step. The iterates are graphed in the x-y plane and printed out in table form. This is an introduction to the idea of prisoners/escapees in iterated functions and the calculation of fractal Julia sets.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Geometry, Graphs, Number and Operations

Related Topics: complex number, coordinate plane, coordinate system, exponents, fractals, functions, graph, infinity, iteration, julia set, mandelbrot set, recursion, recursive functions, sets