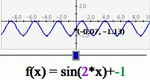Aligned Resources

Explores derivatives and the idea of infinity using a geometric interpretation of slope.

Audiences: Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus

Related Topics: calculus, derivative, differentiate, function properties, graph, linear equations, linear functions, slides, slope, tangent

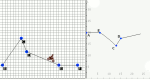
Enter a set of data points, then derive a function to fit those points. Manipulate the function on a coordinate plane using slider bars. Learn how each constant and coefficient affects the resulting graph.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Graphs, Modeling, Statistics, Trigonometry

Related Topics: algebra, cartesian coordinate, coordinate, coordinate plane, coordinate system, cosecant, cosine, cotangent, curve fitting, data plot, deviations, exponential, exponents, function properties, functions, graph, grouping, integers, intervals, inverse, linear equations, linear functions, logarithm, multiplication, parabola, polynomial, positive part of the operand, pre-calculus, range, secant, sine, slope, tangent, trigonometry

View the graph and the equation of the line tangent to any function at any point on the function.

Audiences: Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus

Related Topics: calculus, cartesian coordinate, coordinate plane, derivative, differentiate, function properties, graph, linear equations, slides, slope, tangent

A more advanced version of Slope Slider, this activity allows the manipulation of the constants and coefficients in any function thereby encouraging the user to explore the effects on the graph of the function by changing those numbers.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus, Graphs, Trigonometry

Related Topics: algebra, bell curve, calculus, cartesian coordinate, coordinate plane, coordinate system, cosine, decimals, exponential, flyer, function properties, functions, graph, intervals, inverse, linear equations, linear functions, lines, logarithm, parabola, polynomial, pre-calculus, range, sine, slope, tangent, translation, trigonometry

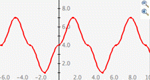
Students can create graphs of functions entered as algebraic expressions -- similar to a graphing calculator.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus, Graphs, Trigonometry

Related Topics: algebra, calculus, cartesian coordinate, coordinate plane, cosecant, cosine, cotangent, functions, graph, inverse, linear equations, linear functions, logarithm, parabola, polynomial, positive part of the operand, pre-calculus, range, secant, sine, slope, tangent, trigonometry

Create graphs of functions and sets of ordered pairs on the same coordinate plane. This is like a graphing calculator with advanced viewing options.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus, Graphs, Statistics, Trigonometry

Related Topics: algebra, calculus, cartesian coordinate, coordinate, coordinate plane, coordinate system, cosecant, cosine, cotangent, curve fitting, data plot, exponential, exponents, function properties, functions, graph, graph theory, intervals, linear equations, linear functions, logarithm, parabola, polynomial, positive part of the operand, pre-calculus, range, secant, sine, slope, tangent, trigonometry

This applet allows the user to make observations about the relationship between speed and position and how both of these are affected by initial velocity and the incline on which the biker is traveling.

Audiences: Grades 9-12, Undergraduate

Primary Subjects: Algebra, Modeling

Related Topics: algebra, distance, functions, graph, parabola, pre-calculus, scale, simulation

Enter a set of data points and a function or multiple functions, then manipulate those functions to fit those points. Manipulate the function on a coordinate plane using slider bars. Learn how each constant and coefficient affects the resulting graph.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus, Modeling, Statistics, Trigonometry

Related Topics: algebra, calculus, cartesian coordinate, coordinate, coordinate plane, coordinate system, cosecant, cosine, cotangent, curve fitting, data plot, deviations, flyer, function properties, functions, graph, linear functions, logarithm, parabola, polynomial, pre-calculus, secant, sine, slides, slope, squares, tangent, trigonometry

Graph recursive functions by defining f(0)=C and defining f(n) based on f(n-1).

Audiences: Grades 9-12, Undergraduate

Primary Subjects: Algebra, Discrete, Graphs, Number and Operations

Related Topics: algebra, arithmetic sequences, cartesian coordinate, coordinate plane, functions, geometric sequences, graph, iteration, recursion, recursive functions, sequences

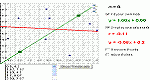
Plot a bivariate data set, determine the line of best fit for their data, and then check the accuracy of your line of best fit.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus, Graphs, Statistics

Related Topics: calculus, cartesian coordinate, coordinate plane, correlation, curve fitting, data plot, deviations, residual, statistics

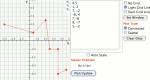
Graph ordered pairs and customize the graph title and axis labels. Points are connected from left to right, rather than being connected in the order they are entered.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Graphs, Statistics

Related Topics: cartesian coordinate, coordinate, coordinate plane, data plot, graph, statistics

Plot ordered pairs of numbers, either as a scatter plot or with the dots connected. Points are connected from right to left, rather than being connected in the order they are entered.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Algebra, Geometry, Graphs, Statistics

Related Topics: cartesian coordinate, coordinate plane, coordinate system, data plot, decimals, graph

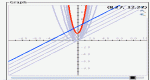
Similar to other "flyers", Slope Slider uses slider bars to explore the effect of the multiplier and constant on a linear function of the form f(x)=mx+b. Explore the relationship between slope and intercept in the Cartesian coordinate system.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Algebra, Graphs

Related Topics: algebra, cartesian coordinate, coordinate plane, decimals, flyer, fractions, function properties, functions, graph, intercept, linear equations, linear functions, slides, slope