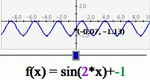Investigation Four: Patterns and Rules

Introduces students to arithmetic and geometric sequences. Students explore further through producing sequences by varying the starting number, multiplier, and add-on.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Algebra, Discrete, Geometry, Number and Operations

Related Topics: addition, arithmetic, arithmetic sequences, geometric sequences, graph, iteration, linear functions, multiplication, multiplier, negative number, pattern, positive number, pre-calculus, recursion, recursive functions, sequences

Introduces students to the idea of finding number patterns in the generation of several different types of fractals.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: area, arithmetic, chaos, decimals, dimension, fractals, fractions, geometric sequences, geometry, graph, iteration, length, lines, pattern, pythagorean theorem, rectangles, recursion, segment, self-similarity, sequences, surface area, symmetry, triangle

Shows students that number patterns exist in the Pascal's Triangle, and reinforces student's ability to identify patterns.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: arithmetic, binomial, chaos, coefficient, combinatorics, decimals, dimension, division, divisors, factors, fractals, fractions, geometric sequences, geometry, infinity, integers, iteration, length, lines, multiples, multiplication, pascal's triangle, pattern, probability, rectangles, recursion, remainders, segment, self-similarity, sequences, surface area, symmetry, triangle, whole numbers

Students learn to identify a variety of patterns using sequences and tessellations.

Audiences: Grades 3-5, Grades 6-8

Primary Subjects: Discrete, Geometry, Number and Operations

Related Topics: addition, arithmetic, arithmetic sequences, geometric sequences, hexagon, iteration, multiplication, pattern, planes, polygon, recursion, recursive functions, sequences, squares, symmetry, tessellations, triangle

Enter a set of data points, then derive a function to fit those points. Manipulate the function on a coordinate plane using slider bars. Learn how each constant and coefficient affects the resulting graph.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Graphs, Modeling, Statistics, Trigonometry

Related Topics: algebra, cartesian coordinate, coordinate, coordinate plane, coordinate system, cosecant, cosine, cotangent, curve fitting, data plot, deviations, exponential, exponents, function properties, functions, graph, grouping, integers, intervals, inverse, linear equations, linear functions, logarithm, multiplication, parabola, polynomial, positive part of the operand, pre-calculus, range, secant, sine, slope, tangent, trigonometry

Create your own fractals by drawing a "line deformation rule" and stepping through the generation of a geometric fractal. Parameters: Grid type, number of bending points on the line.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Geometry, Number and Operations

Related Topics: chaos, fractals, geometric sequences, iteration, pattern, pre-calculus, recursion, scale, self-similarity, sequences, symmetry, transformation

Students investigate very simple functions by trying to guess the algebraic form from inputs and outputs. Function Machine is one of the Interactivate assessment explorers.

Audiences: Grades 6-8, Grades 9-12

Primary Subjects: Algebra, Discrete

Related Topics: addition, algebra, arithmetic, assessment, function machine, functions, input, integers, linear equations, multiplication, output, subtraction

Students can create graphs of functions entered as algebraic expressions -- similar to a graphing calculator.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus, Graphs, Trigonometry

Related Topics: algebra, calculus, cartesian coordinate, coordinate plane, cosecant, cosine, cotangent, functions, graph, inverse, linear equations, linear functions, logarithm, parabola, polynomial, positive part of the operand, pre-calculus, range, secant, sine, slope, tangent, trigonometry

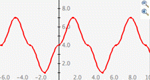
Create graphs of functions and sets of ordered pairs on the same coordinate plane. This is like a graphing calculator with advanced viewing options.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Calculus, Graphs, Statistics, Trigonometry

Related Topics: algebra, calculus, cartesian coordinate, coordinate, coordinate plane, coordinate system, cosecant, cosine, cotangent, curve fitting, data plot, exponential, exponents, function properties, functions, graph, graph theory, intervals, linear equations, linear functions, logarithm, parabola, polynomial, positive part of the operand, pre-calculus, range, secant, sine, slope, tangent, trigonometry

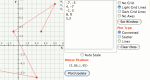
Plot ordered pairs on the graph, and they will be connected in the order that they are input. This enables you to decide how the pairs should be connected, rather than having the computer connect them from left to right.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Algebra, Geometry, Graphs, Statistics

Related Topics: cartesian coordinate, coordinate, coordinate plane, coordinate system, data plot, graph, lines, vertical line test

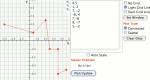
Plot ordered pairs of numbers, either as a scatter plot or with the dots connected. Points are connected from right to left, rather than being connected in the order they are entered.

Audiences: Grades 3-5, Grades 6-8, Grades 9-12

Primary Subjects: Algebra, Geometry, Graphs, Statistics

Related Topics: cartesian coordinate, coordinate plane, coordinate system, data plot, decimals, graph

Enter two complex numbers (z and c) as ordered pairs of real numbers, then click a button to iterate step by step. The iterates are graphed in the x-y plane and printed out in table form. This is an introduction to the idea of prisoners/escapees in iterated functions and the calculation of fractal Julia sets.

Audiences: Grades 6-8, Grades 9-12, Undergraduate

Primary Subjects: Algebra, Geometry, Graphs, Number and Operations

Related Topics: complex number, coordinate plane, coordinate system, exponents, fractals, functions, graph, infinity, iteration, julia set, mandelbrot set, recursion, recursive functions, sets