What is Equivalent Fractions Pointer?
This activity allows the user to visually experiment with the relationship between the values of equivalent fractions and areas within a square or a circle.
The activity provides the user with a fraction, its equivalent representation as a shaded portion of a square or circle, and a number line. Also displayed are two empty squares or circles. Each shape is connected to a point on the number line corresponding to the fraction represented by the shape. The goal is for the user to add and color sections in the blank shapes to create fractions equal to the original computer generated fraction.
How Do I Use This Activity?
This activity helps the user to visualize the concept of equivalent fractions. The user has to illustrate two fractions which have the same value as a third given fraction, but with different numerators and denominators.
Controls and Output
-
You can choose from two different types of fraction diagrams in the
Choose Shape pull-down menu: square or circle. If you change shapes in the middle of a problem, all your
work to that point will be lost.
-
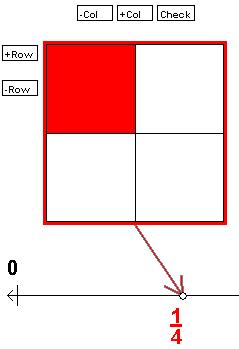
Below is an example of the square fraction diagram. You can change the number of columns by
using the
-Col button to remove columns and the
+Col button to add columns. You can change the number of rows by using the
+Row and
-Row buttons. To color a section in the diagram, click the section. You can un-color a section
by clicking it again.
-
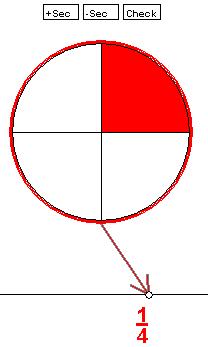
Below is an example of the circular fraction diagram. You can change the number of sections
by using the
+Sec button to add sections and the
-Sec button to remove sections. To color a section in the diagram, click the section. You can
un-color a section by clicking it again.
- You can choose to create one or two equivalent fractions by selecting "one" or "two" from the Number of Fractions drop down menu.
- For each equivalent fraction, you should make the colored part of the diagram equal to the given fraction. Then click the Check button for each diagram to check your answer. If the diagram is correct, the word "Correct" will be printed on top of the diagram; if it isn't correct, the word "Incorrect" will be printed instead. If your answer is incorrect, you can try again. If you repeat the given fraction or a fraction you've already made with the same numerator and denominator the word "Repeat" will be printed.
-
At any time during the activity, you can click the
Start Over button or the
Next Question button. The
Start Over button will restart the current question from the very beginning. The
Next Question button will set up a new question.

- This activity will automatically record how successful you are at answering the questions. To view the score, press the Show Score button at the bottom of the activity and a pop-up window will appear with the scoreboard. To close this pop-up window press the Close button or click back on the main window.
- To pause the scoring, press the Active button at the bottom of the screen and it will change to a Paused button. To resume scoring, press the Paused button.
- To reset the scoreboard, open the scoreboard using the Show Score button and then press the Reset button.
Description
This activity allows the user to visually experiment with the relationship between the value of fractions and areas within a square or a circle. This activity would work well in groups of two to four for about 30 minutes if you use the exploration questions.
Place in Mathematics Curriculum
This activity can be used to:
- Practice simplifying fractions
- Compare fractions and order them on a number line
- Develop the concept of equivalent fractions.
Standards Addressed
Grade 3
-
Numeration
- The student demonstrates conceptual understanding of simple fractions with denominators 2, 3, 4, or 10.
Grade 4
-
Numeration
- The student demonstrates conceptual understanding of fractions with denominators 2 through 12.
Grade 5
-
Numeration
- The student demonstrates conceptual understanding of positive fractions with denominators 1 through 12 and 100 with proper and mixed numbers and benchmark percents (10%, 25%, 50%, 75%, 100%).
Grade 6
-
Numeration
- The student demonstrates conceptual understanding of fractions (proper or mixed numbers), decimals, percents (whole number), or integers.
- The student demonstrates conceptual understanding of fractions, mixed numbers, or percents.
Grade 7
-
Numeration
- The student demonstrates conceptual understanding of rational numbers (fractions, decimals, percents, or integers).
- The student demonstrates conceptual understanding of positive fractions, decimals, or percents.
Grade 8
-
Numeration
- The student demonstrates conceptual understanding of real numbers.
- The student demonstrates conceptual understanding of rational numbers (fractions, decimals, or percents including integers).
Grade 9
-
Numeration
- The student demonstrates conceptual understanding of real numbers.
Grade 10
-
Numeration
- The student demonstrates conceptual understanding of real numbers.
Third Grade
-
Number and Operations-Fractions
- Develop understanding of fractions as numbers.
Fifth Grade
-
Number and Operations-Fractions
- Use equivalent fractions as a strategy to add and subtract fractions.
Grades 6-8
-
Numbers and Operations
- Understand meanings of operations and how they relate to one another
Grade 4
-
Number and Operations, Measurement, Geometry, Data Analysis and Probability, Algebra
- COMPETENCY GOAL 1: The learner will read, write, model, and compute with non-negative rational numbers.
Grade 5
-
Number and Operations, Measurement, Geometry, Data Analysis and Probability, Algebra
- COMPETENCY GOAL 1: The learner will understand and compute with non-negative rational numbers.
Grade 6
-
Number and Operations, Measurement, Geometry, Data Analysis and Probability, Algebra
- COMPETENCY GOAL 1: The learner will understand and compute with rational numbers.
Grade 7
-
Number and Operations, Measurement, Geometry, Data Analysis and Probability, Algebra
- COMPETENCY GOAL 1: The learner will understand and compute with rational numbers.
Grade 3
-
Geometry and Spatial Reasoning
- 10. The student recognizes that a line can be used to represent numbers and fractions and their properties and relationships. The student is expected to locate and name points on a number line using whole numbers and fractions, including halves and fourths.
-
Number, Operation, and Quantitative Reasoning
- 2. The student uses fraction names and symbols (with denominators of 12 or less) to describe fractional parts of whole objects or sets of objects.
Grade 4
-
Geometry and Spatial Reasoning
- 10. The student recognizes the connection between numbers and their properties and points on a line. The student is expected to locate and name points on a number line using whole numbers, fractions such as halves and fourths, and decimals such as tenths.
-
Number, Operation, and Quantitative Reasoning
- 2. The student describes and compares fractional parts of whole objects or sets of objects.
3rd Grade
-
Number and Number Sense
- 3.05a The student will divide regions and sets to represent a fraction;
- 3.05b The student will name and write the fractions represented by a given model (area/region, length/measurement, and set). Fractions (including mixed numbers) will include halves, thirds, fourths, eighths, and tenths.
- 3.06 The student will compare the numerical value of two fractions having like and unlike denominators, using concrete or pictorial models involving areas/regions, lengths/measurements, and sets.
4th Grade
-
Number and Number Sense
- 4.3 The student will compare the numerical value of fractions (with like and unlike denominators) having denominators of 12 or less, using concrete materials.
Textbooks Aligned
Grade Six
-
Bits and Pieces I
- Investigation One: Fund-Raising Fractions
- Investigation Two: Comparing Fractions
-
Bits and Pieces II
- Investigation Three: Estimating with Fractions and Decimals
Book 1
-
Module 2 - Patterns and Designs
- Section 3: Equivalent Fractions
-
Module 5 - Creating Things
- Section 1: Fraction Number Sense
Book 2
-
Module 3 - A Universal Language
- Section 2: Comparing Fractions, Adding and Subtracting Fractions
6th
-
Module 1 - Patterns and Problem Solving
- Section 5: Equivalent Fractions
7th
-
Module 3 - Codes
- Section 3: Fractions
Book 1
-
From Zero to One and Beyond
- Lesson 2: Fraction Circles
Book 2
-
Buyer Beware
- Lesson 5: Dancing Ratios
- Lesson 6: Which Brand Has the Most Chocolate?
Grade 5
-
Some of the Parts
- Fractions
- Relationships between Fractions
- Operations with Fractions
Grade 6
-
Fraction Times
- Operations with Fractions
- Fraction/Percent/Decimal/Ration Relationships
Grade 7
-
Cereal Numbers
- Fractions
Be Prepared to
- give implicit directions on what they are to do. For example "Today we are going to compare equivalent fractions and use the number line."
- discuss different methods to mentally approximate fractions.
- discuss reducing fractions and equivalent fractions.