What is Possible or Not?
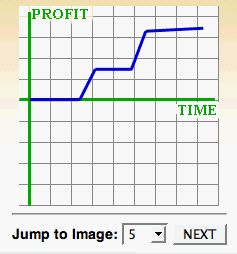
This activity allows the user to look at graphs of functions for real life situations, and asks them to decide if the graph is possible (makes sense) in context.
The main idea here is that graphs showing the relationships between two quantities, like time and speed for an aircraft, have certain laws that they have to follow. For example, speed doesn't change instantly -- if we are going 10 mph and then later we have sped up to 20 mph, sometime in the middle we have to be going 15 mph.
The graphs shown here all relate two common everyday quantities. Judge which are possible in context and which are not.
How Do I Use This Activity?
This activity allows the user to view graphs of potential real life situations and to decide if they represent functions.
Controls and Output
- Select the image to view (numbers 1 through 10), and the graph will automatically update. You can also click the Next button to load the next image. For each graph, determine whether it is possible or not.
Description
This activity allows the user to look at graphs of functions for real life situations, and asks them to decide if the graph is possible (makes sense) in context. This activity would work well in same ability groups of two to four for about fifteen to twenty minutes if you use the exploration questions and five to ten minutes otherwise.
Place in Mathematics Curriculum
This activity can be used to:
- practice students' function skills
- practice students' deductive reasoning skills
- practice students' graphing skills
Standards Addressed
Grade 9
-
Functions and Relationships
- The student demonstrates conceptual understanding of functions, patterns, or sequences including those represented in real-world situations.
- The student demonstrates algebraic thinking.
Grade 10
-
Functions and Relationships
- The student demonstrates conceptual understanding of functions, patterns, or sequences including those represented in real-world situations.
- The student demonstrates algebraic thinking.
Functions
-
Interpreting Functions
- Understand the concept of a function and use function notation
- Interpret functions that arise in applications in terms of the context
- Analyze functions using different representations
-
Linear, Quadratic, and Exponential Models
- Interpret expressions for functions in terms of the situation they model
Grades 6-8
-
Algebra
- Represent and analyze mathematical situations and structures using algebraic symbols
Elementary Algebra
-
Elementary Algebra
- Standard EA-1: The student will understand and utilize the mathematical processes of problem solving, reasoning and proof, communication, connections, and representation.
- Standard EA-3: The student will demonstrate through the mathematical processes an understanding of relationships and functions.
Algebra I
-
Linear Functions
- 6. The student understands the meaning of the slope and intercepts of the graphs of linear functions and zeros of linear functions and interprets and describes the effects of changes in parameters of linear functions in real-world and mathematical situations.
Secondary
-
Algebra II
- AII.08 The student will recognize multiple representations of functions (linear, quadratic, absolute value, step, and exponential functions) and convert between a graph, a table, and symbolic form. A transformational approach to graphing will be employed through the use of graphing calculators.
- AII.09 The student will find the domain, range, zeros, and inverse of a function; the value of a function for a given element in its domain; and the composition of multiple functions. Functions will include exponential, logarithmic, and those that have domains and ranges that are limited and/or discontinuous. The graphing calculator will be used as a tool to assist in investigation of functions.
- AII.8
- AII.9
Textbooks Aligned
Grade Seven
-
Variables and Patterns
- Investigation One: Variables and Coordinate Graphs
- Investigation Two: Graphing Change
Book 1
-
Module 4 - Mind Games
- Section 5: Graphing on the Coordinate Grid
- Section 5: Expressions and Equations Multiples
-
Module 6 - Comparisons and Predictions
- Section 3: Using Ratios
-
Module 7 - Wonders of the World
- Section 6: Graphing Ordered Pairs
-
Module 8 - Our Environment
- Section 2: Representing Population Data
Book 2
-
Module 2 - Search and Rescue
- Section 2: Coordinate Graphing of Integers
- Section 4: Modeling Functions
-
Module 5 - Recreation
- Section 2: Scatter Plots
-
Module 7 - Health and Wellness
- Section 1: Formulas and Graphs
- Section 1: Misleading Graphs
Book 3
-
Module 2 - At the Mall
- Section 1: Percent
-
Module 3 - The Mystery of Blacktail Canyon
- Section 2: Graphing Equations
Book 3
-
Family Portraits
- Lesson 1: Inputs and Outputs
Grade 8
-
Get the Most Out of It
- Word Problems with Two Unknowns
- Systems of Equations
- Graphing Lines
- Graphing Inequalities
- Graphing Hyperbolas
-
Great Expectations
- Variability
- Chance
- Sample Size
- Probability
- Combinations
- Simulations
-
Growth
- Linear functions
- Quadratic Functions
- Cubic Functions
- Exponential Functions
- Sequences
- Growth
Grade 5
-
Figuring All the Angles
- Measuring Angles
- Vectors
- Rectangular Coordinates
- Polar Coordinates
-
Patterns and Symbols
- Variables
- Patterns
- Pattern Rule
-
Picturing Numbers
- Bar Graphs
- Line Graphs
- Pie Charts
- Pictographs
- Describing Data
- Using Data for Arguments
Grade 6
-
Dealing With Data
- Scatter Plots
- Box Plots
- Stem and Leaf Plot
- Histograms
- Mean, Median, Mode and Range
-
Operations
- Operations with Integers
- Plotting Points
- Coordinate Plane
-
Tracking Graphs
- Line Graphs
- Interpreting Graphs
- Looking at a Graph's global features
Grade 7
-
Packages and Polygons
- Geometric Shapes
- Models
- Properties of Regular and Semi-Regular Polyhedra
-
Ups and Downs
- Increasing and Decreasing Functions
- Linear Functions
- Periodic Functions
Be Prepared to
- answer the question "What makes a graph not a function?"
- discuss continuity and the vertical line test